Linear equations in two variables

Key Concepts: Ordered pairs/The Rectangular Coordinate system
Solutions to equations with 2 variables
Linear equations in two variables
Intercepts
Horizontal and Vertical lines

Martin-Gaye sections and practice problems

3.1: 1 – 19 odd, 23 – 51 odd, 55, 57
3.2: 1 – 43 odd, 47, 53, 55
3.3: 1 – 10 all, 11 – 63 odd

Definitions
A solution to an equation with two variables, x and y , is an ordered pair (a,b) where a and b are
real numbers with the property that if x = a and y = b the equation is true. The number a is called
the x-coordinate of the ordered pair and the number b is called the y-coordinate of the ordered pair.

Example 1
What are the x and y coordinates of the ordered pair (9,− 2) . Is the ordered pair a solution to
the equation 3 x + 4 y = 10 + x ?


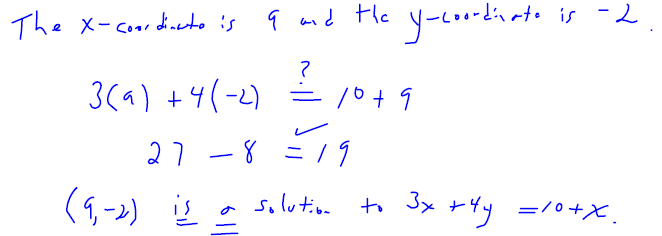
Example 2
What is the ordered pair with a x-coordinate of 5 that satisfies the equation y = 6 x + 4


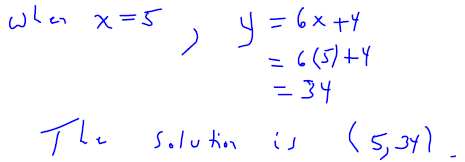
Example 3
What is the ordered pair with a y-coordinate of 5 that satisfies the equation y = 6 x + 4


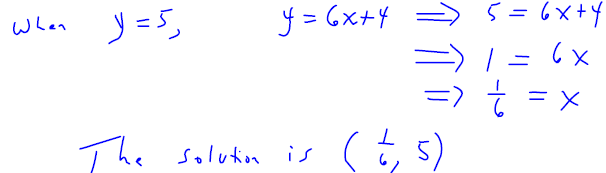
Example 4
Write the missing values into Table 1 so that each implied ordered pair is a solution to the equation
x + y = 4. Plot the four ordered pairs onto Figure 1.

Table 1: x + y = 4

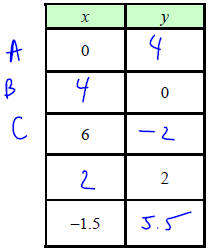Example 5
The sum of the the coordinates of each point on the line in Figure 2 is always the same number.
What is this constant sum?

Example 6
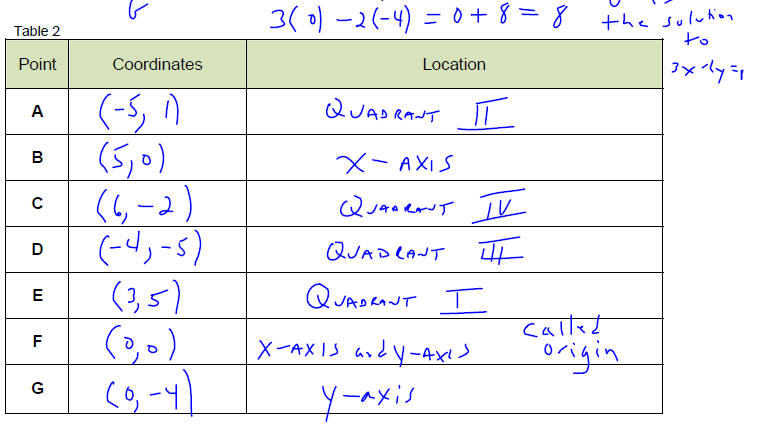
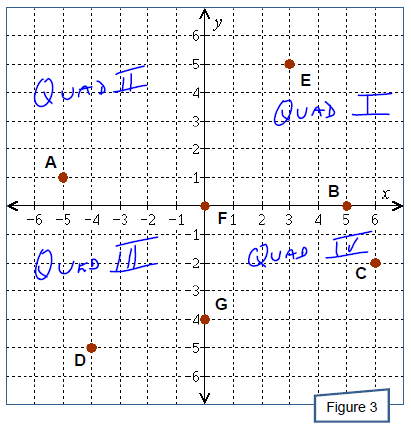
Several points are shown in Figure 3. State the ordered pair associated with each point and where
in the coordinate plane the point lies; assume that both coordinates of each point are intergers.
Which point, B or F, is a solution to the equation 3x − 2 y = 8 ?

Example 7
Plot the points A(−3, 4) , B(−3, −3) , and C(5, 2) onto
Figure 4 and find the area, A, of the resultant triangle.
Assume that the scale on each axis is in centimeters.

Example 8
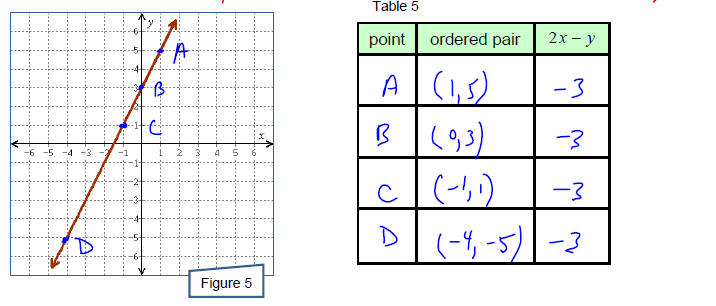
At each point on the line in Figure 5, twice the x-coordinate minus the y-coordinate is always the
same number. What is this common difference?